To make a seven-sided sigil, mark seven points equally spaced around a circle, and then join the points together with a single line which visits each point once and once only. Here are some examples of the shapes you get:



It turns out that there are exactly 39 different shapes you can get – although they can appear rotated or reflected. Seven-sided sigils appear in a number of the Sareoso diagrams.
The seven-sided sigils have their own relationships and characteristics, and can be categorised in different ways. For example, three of the sigils always look the same no matter how they are rotated or reflected. Another 21 have just one axis of symmetry, and the other 15 have no symmetry.
One way of considering the relationships between different sigils is to consider pair-swaps, where you switch two points on a sigil and see what other sigil results. For example:
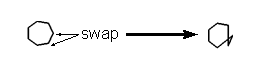
The table below shows all the possible pair swap connections between the sigils.

The table is complex, but there are patterns. For example, looking at the right hand side, we can see that there are:
3 sigils that swap to 3 others (9 swaps in total)
3 sigils that swap to 21 others (63 swaps in total)
3 sigils that swap to 10 others (30 swaps in total
18 sigils that swap to 11 others (198 swaps in total)
12 sigils that swap to 16 other (192 swaps in total)
The pair swaps can be used to further categorise the sigils, as discussed in this article.